These properties will be necessary to solving equations. They should be committed to memory.

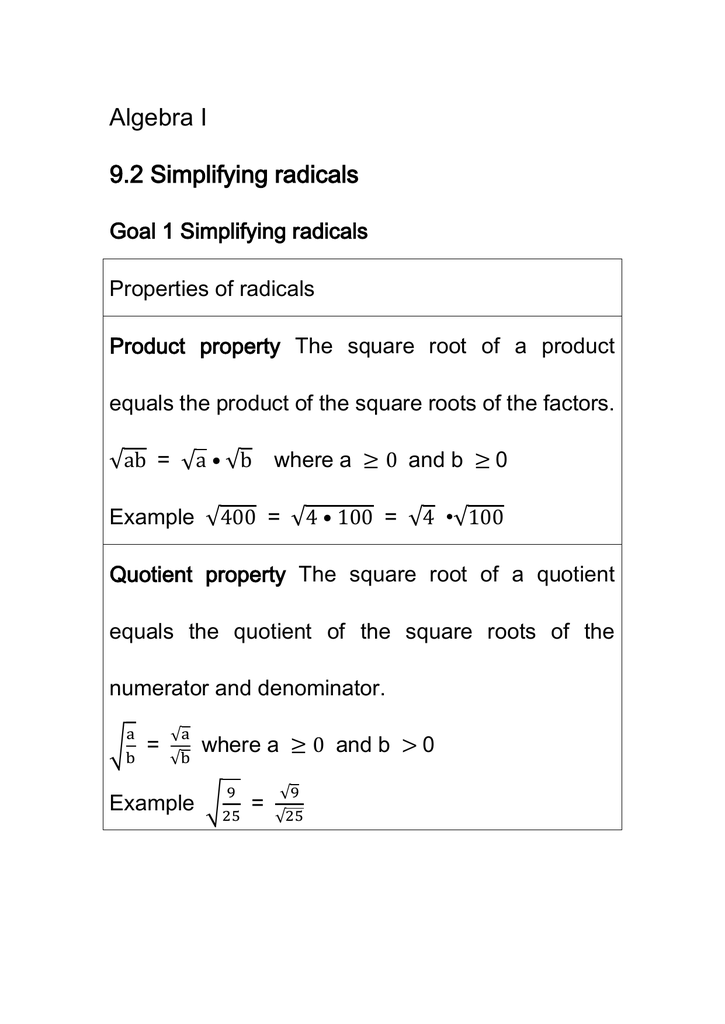
Logarithm of a Quotient You can use the similarity between the properties of exponents and logarithms to find the property for the logarithm of a quotient. With exponents, to multiply two numbers with the same base, you add the exponents. To divide two numbers with the same base, you subtract the exponents.

Quotient Property Khan

Properties of Equality

The first four properties of equality--those that deal with operations--allow us to add, subtract, multiply and divide variables. They also formally express the idea that when we perform the same operations on both sides of an equation, the two sides are still equivalent. In other words, that we can perform the same operation on both sides without changing the values of the variables.

The quotient property of square roots if very useful when you're trying to take the square root of a fraction. This property allows you to split the square root between the numerator and denominator of. Quotient of powers Calculator Get detailed solutions to your math problems with our Quotient of powers step-by-step calculator. Practice your math skills and learn step by step with our math solver. Check out all of our online calculators here! A special case of the Quotient Property is when the exponents of the numerator and denominator are equal, such as an expression like am am a m a m. From earlier work with fractions, we know that 2 2 =117 17 = 1−43 −43 =1 2 2 = 1 17 17 = 1 − 43 − 43 = 1 In words, a number divided by itself is 1 1.

The next four properties formally express the idea that the quantities on each side of the '=' sign mean the same thing as each other, and that is does not matter which quantity is on which side of the '=' sign.

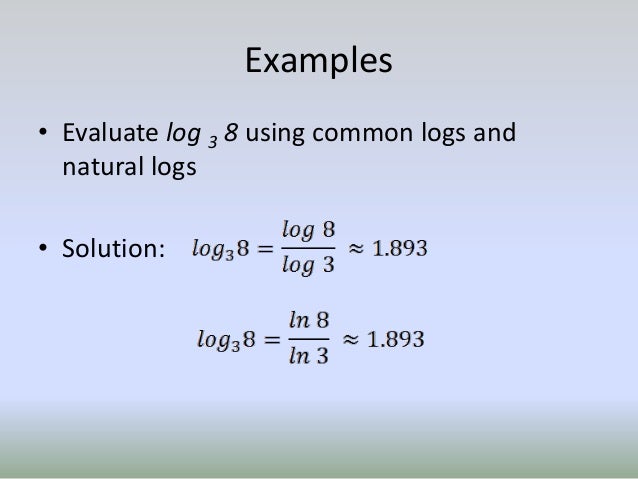
Quotient Property Examples

The final property--the Zero Product Property--is the inverse of the Multiplication Property of Zero learned in Pre-Algebra. The Multiplication Property of Zero states that the product of several numbers, one which is zero, is zero. The Zero Product Property states that if the product of several numbers is zero, then at least one of them must be zero--we cannot multiply non-zero numbers and get zero.

Properties of Operations and Identities

The properties of operations and identities formally express the properties learned in the Operations section of the Pre-Algebra SparkNote, with two additions--the Quotient Property and the Multiplicative Inverse Property. The Quotient Property stems from the fact that we multiply two fractions by multiplying their numerators and then multiplying their denominators. When we multiply a by , the numerator is a×1 = a and the denominator is 1×b = b; thus, the result is . The Quotient Property shows that multiplying by a fraction whose numerator is 1 is the same as dividing by the denominator of that fraction, and that dividing by a number is the same as putting the divisor in the denominator of a fraction whose numerator is the given number.

Quotient Properties Of Exponents

We can see how the Multiplicative Inverse Property works using the Quotient Property. We want to find a number that yields 1 when multiplied by a, or that yields when multiplied by a, since = 1. Thus, we have a× = , where represents the fraction we are trying to find. Using the Quotient Property, we can replace b with a to yield the desired equation -- thus, has 1 in the numerator and a in the denominator: = . The number is called the reciprocal or multiplicative inverse of a.